Kepler’s First Law

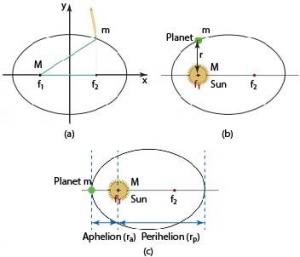
The orbit of each planet about the sun is an ellipse with the sun at one focus, as shown in Figure 7.2. The planet’s closest approach to the sun is called perihelion and its farthest distance from the sun is called aphelion.

Figure 7.2 (a) An ellipse is a closed curve such that the sum of the distances from a point on the curve to the two foci (f₁ and f₂) is constant. (b) For any closed orbit, m follows an elliptical path with M at one focus. (c) The perihelion (rp) is the closest distance between the planet and the sun, while the aphelion (ra) is the farthest distance from the sun.

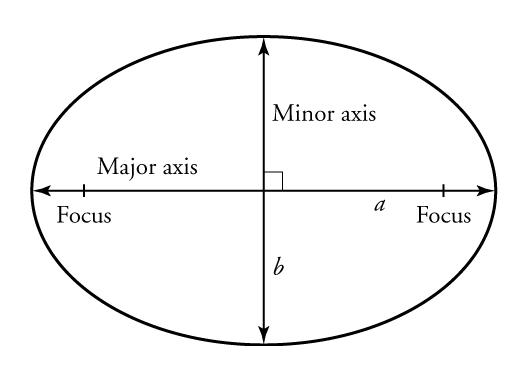
If you know the aphelion (r_a) and perihelion (r_p) distances, then you can calculate the semi-major axis (a) and semi-minor axis (b).

Figure 7.3 You can draw an ellipse as shown by putting a pin at each focus, and then placing a loop of string around a pen and the pins and tracing a line on the paper.

Kepler’s Second Law

Each planet moves so that an imaginary line drawn from the sun to the planet sweeps out equal areas in equal times, as shown in Figure 7.4.

Figure 7.4 The shaded regions have equal areas. The time for m to go from A to B is the same as the time to go from C to D and from E to F. The mass m moves fastest when it is closest to M. Kepler’s second law was originally devised for planets orbiting the sun, but it has broader validity.

Kepler’s Third Law

The ratio of the periods squared of any two planets around the sun is equal to the ratio of their average distances from the sun cubed. In equation form, this is

where T is the period (time for one orbit) and r is the average distance (also called orbital radius). This equation is valid only for comparing two small masses orbiting a single large mass. Most importantly, this is only a descriptive equation; it gives no information about the cause of the equality.

Links To Physics

History: Ptolemy vs. Copernicus

Before the discoveries of Kepler, Copernicus, Galileo, Newton, and others, the solar system was thought to revolve around Earth as shown in Figure 7.5 (a). This is called the Ptolemaic model, named for the Greek philosopher Ptolemy who lived in the second century AD. The Ptolemaic model is characterized by a list of facts for the motions of planets, with no explanation of cause and effect. There tended to be a different rule for each heavenly body and a general lack of simplicity.

Figure 7.5 (b) represents the modern or Copernican model. In this model, a small set of rules and a single underlying force explain not only all planetary motion in the solar system, but also all other situations involving gravity. The breadth and simplicity of the laws of physics are compelling.

Figure 7.5 (a) The Ptolemaic model of the universe has Earth at the center with the moon, the planets, the sun, and the stars revolving about it in complex circular paths. This geocentric (Earth-centered) model, which can be made progressively more accurate by adding more circles, is purely descriptive, containing no hints about the causes of these motions. (b) The Copernican heliocentric (sun-centered) model is a simpler and more accurate model.

Nicolaus Copernicus (1473–1543) first had the idea that the planets circle the sun, in about 1514. It took him almost 20 years to work out the mathematical details for his model. He waited another 10 years or so to publish his work. It is thought he hesitated because he was afraid people would make fun of his theory. Actually, the reaction of many people was more one of fear and anger. Many people felt the Copernican model threatened their basic belief system. About 100 years later, the astronomer Galileo was put under house arrest for providing evidence that planets, including Earth, orbited the sun. In all, it took almost 300 years for everyone to admit that Copernicus had been right all along.

Grasp Check

Explain why Earth does actually appear to be the center of the solar system.

1. Earth appears to be the center of the solar system because Earth is at the center of the universe, and everything revolves around it in a circular orbit.
2. Earth appears to be the center of the solar system because, in the reference frame of Earth, the sun, moon, and planets all appear to move across the sky as if they were circling Earth.
3. Earth appears to be at the center of the solar system because Earth is at the center of the solar system and all the heavenly bodies revolve around it.
4. Earth appears to be at the center of the solar system because Earth is located at one of the foci of the elliptical orbit of the sun, moon, and other planets.

Virtual Physics

Acceleration

This simulation allows you to create your own solar system so that you can see how changing distances and masses determines the orbits of planets. Click Help for instructions.

Figure 7.6 Click here for the simulation

Grasp Check

When the central object is off center, how does the speed of the orbiting object vary?

1. The orbiting object moves fastest when it is closest to the central object and slowest when it is farthest away.
2. The orbiting object moves slowest when it is closest to the central object and fastest when it is farthest away.
3. The orbiting object moves with the same speed at every point on the circumference of the elliptical orbit.
4. There is no relationship between the speed of the object and the location of the planet on the circumference of the orbit.

Kepler’s First Law

Refer back to this figure (a). Notice which distances are constant. The foci are fixed, so distance $\overline{f_1{f}_2}$ is a constant. The definition of an ellipse states that the sum of the distances $\overline{f_{1}m}+\overline{m{f}_2}$ is also constant. These two facts taken together mean that the perimeter of triangle $\Delta f_{1}m{f}_2$ must also be constant. Knowledge of these constants will help you determine positions and distances of objects in a system that includes one object orbiting another.

Kepler’s Second Law

Refer back to this figure. The second law says that the segments have equal area and that it takes equal time to sweep through each segment. That is, the time it takes to travel from A to B equals the time it takes to travel from C to D, and so forth. Velocity v equals distance d divided by time t: $v=d/t$. Then, $t=d/v$, so distance divided by velocity is also a constant. For example, if we know the average velocity of Earth on June 21 and December 21, we can compare the distance Earth travels on those days.

The degree of elongation of an elliptical orbit is called its eccentricity (e). Eccentricity is calculated by dividing the distance f from the center of an ellipse to one of the foci by half the long axis a.

When $e=0$, the ellipse is a circle.

The area of an ellipse is given by $A=\pi ab$, where b is half the short axis. If you know the axes of Earth’s orbit and the area Earth sweeps out in a given period of time, you can calculate the fraction of the year that has elapsed.

Worked Example

Kepler’s Second Law

Figure 7.7 shows the major and minor axes of an ellipse. The semi-major and semi-minor axes are half of these, respectively.

Figure 7.7 The major axis is the length of the ellipse, and the minor axis is the width of the ellipse. The semi-major axis is half the major axis, and the semi-minor axis is half the minor axis.

Earth’s orbit is slightly elliptical, with a semi-major axis of 152 million km and a semi-minor axis of 147 million km. If Earth’s period is 365.26 days, what area does an Earth-to-sun line sweep past in one day?

Strategy

Each day, Earth sweeps past an equal-sized area, so we divide the total area by the number of days in a year to find the area swept past in one day. For total area use $A=\pi ab$. Calculate A, the area inside Earth’s orbit and divide by the number of days in a year (i.e., its period).

Solution

7.2$$\begin{array}{ccc}\hfill \text{area per day}& =& \frac{\text{total area}}{\text{total number of days}}\hfill \\ & =& \frac{\pi ab}{\text{365 d}}\hfill \\ & =& \frac{\pi \left(1.47\times {10}^8\text{ km}\right)\left(1.52\times {10}^3\text{ km}\right)}{\text{365 d}}\hfill \\ & =& 1.92\times {10}^{14}{\text{ km}}^2/\text{d}\hfill \end{array}$$

The area swept out in one day is thus $1.92\times {10}^{14}{\text{km}}^{\text{2}}$.

Discussion

The answer is based on Kepler’s law, which states that a line from a planet to the sun sweeps out equal areas in equal times.

Kepler’s Third Law

Kepler’s third law states that the ratio of the squares of the periods of any two planets (T₁, T₂) is equal to the ratio of the cubes of their average orbital distance from the sun (r₁, r₂). Mathematically, this is represented by

From this equation, it follows that the ratio r³/T² is the same for all planets in the solar system. Later we will see how the work of Newton leads to a value for this constant.