Learning Objectives

By the end of this section, you will be able to do the following:

Explain reflection from mirrors, describe image formation as a consequence of reflection from mirrors, apply ray diagrams to predict and interpret image and object locations, and describe applications of mirrors
Perform calculations based on the law of reflection and the equations for curved mirrors

Section Key Terms
angle of incidence	angle of reflection	central axis
concave mirror	convex mirror	diffused
focal length	focal point	geometric optics
law of reflection	law of refraction	ray
real image	specular	virtual image

Characteristics of Mirrors

There are three ways, as shown in Figure 16.2, in which light can travel from a source to another location. It can come directly from the source through empty space, such as from the Sun to Earth. Light can travel to an object through various media, such as air and glass. Light can also arrive at an object after being reflected, such as by a mirror. In all these cases, light is modeled as traveling in a straight line, called a ray. Light may change direction when it encounters the surface of a different material (such as a mirror) or when it passes from one material to another (such as when passing from air into glass). It then continues in a straight line—that is, as a ray. The word ray comes from mathematics. Here it means a straight line that originates from some point. It is acceptable to visualize light rays as laser rays (or even science fiction depictions of ray guns).

In view (a), a cartoon image of Earth contains a house superimposed over North America. The Sun is included above and to the right of Earth. An arrow points from the Sun’s rays to a series of lines, which represent Earth’s atmosphere, directly above the Earth. In view (b), a woman is looking out of a window at her car. Arrows show the Sun’s rays bouncing off the car and traveling as light toward the woman.

Figure 16.2 Three methods for light to travel from a source to another location are shown. (a) Light reaches the upper atmosphere of Earth by traveling through empty space directly from the source (the Sun). (b) This light can reach a person in one of two ways. It can travel through a medium, such as air or glass, and typically travels from one medium to another. It can also reflect from an object, such as a mirror.

Because light moves in straight lines, that is, as rays, and changes directions when it interacts with matter, it can be described through geometry and trigonometry. This part of optics, described by straight lines and angles, is therefore called geometric optics. There are two laws that govern how light changes direction when it interacts with matter: the law of reflection, for situations in which light bounces off matter; and the law of refraction, for situations in which light passes through matter. In this section, we consider the geometric optics of reflection.

Whenever we look into a mirror or squint at sunlight glinting from a lake, we are seeing a reflection. How does the reflected light travel from the object to your eyes? The law of reflection states: The angle of reflection, $θ_{r}$ , equals the angle of incidence, $θ_{i}$ . This law governs the behavior of all waves when they interact with a smooth surface, and therefore describe the behavior of light waves as well. The reflection of light is simplified when light is treated as a ray. This concept is illustrated in Figure 16.3, which also shows how the angles are measured relative to the line perpendicular to the surface at the point where the light ray strikes it. This perpendicular line is also called the normal line, or just the normal. Light reflected in this way is referred to as specular (from the Latin word for mirror: speculum).

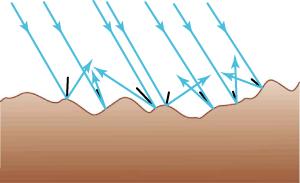
We expect to see reflections from smooth surfaces, but Figure 16.4, illustrates how a rough surface reflects light. Because the light is reflected from different parts of the surface at different angles, the rays go in many different directions, so the reflected light is diffused. Diffused light allows you to read a printed page from almost any angle because some of the rays go in different directions. Many objects, such as people, clothing, leaves, and walls, have rough surfaces and can be seen from many angles. A mirror, on the other hand, has a smooth surface and reflects light at specific angles.

A smooth, flat reflective surface is shown. A dotted line drops from the top of the figure and ends when it reaches the smooth surface. This line is perpendicular to the surface; there is a 90° angle between the surface and the perpendicular line. To the left of the perpendicular line, a ray (called an 'incident ray' strikes the surface at a 45° angle. This ray—the 'reflective ray'—is reflected off the surface to the right of the perpendicular line, again at a 45° angle. The angle between t

Figure 16.3 The law of reflection states that the angle of reflection, θ_r, equals the angle of incidence, θ_i. The angles are measured relative to the line perpendicular to the surface at the point where the ray strikes the surface. The incident and reflected rays, along with the normal, lie in the same plane.

A rough surface, such as the ground, is shown. Multiple rays are striking the uneven surface and are bouncing off the ground in different directions.

Figure 16.4 Light is diffused when it reflects from a rough surface. Here, many parallel rays are incident, but they are reflected at many different angles because the surface is rough.

When we see ourselves in a mirror, it appears that our image is actually behind the mirror. We see the light coming from a direction determined by the law of reflection. The angles are such that our image is exactly the same distance behind the mirror, d_i, as the distance we stand away from the mirror, d_o. Although these mirror images make objects appear to be where they cannot be (such as behind a solid wall), the images are not figments of our imagination. Mirror images can be photographed and videotaped by instruments and look just as they do to our eyes, which are themselves optical instruments. An image in a mirror is said to be a virtual image, as opposed to a real image. A virtual image is formed when light rays appear to diverge from a point without actually doing so.

Figure 16.5 helps illustrate how a flat mirror forms an image. Two rays are shown emerging from the same point, striking the mirror, and reflecting into the observer’s eye. The rays can diverge slightly, and both still enter the eye. If the rays are extrapolated backward, they seem to originate from a common point behind the mirror, allowing us to locate the image. The paths of the reflected rays into the eye are the same as if they had come directly from that point behind the mirror. Using the law of reflection—the angle of reflection equals the angle of incidence—we can see that the image and object are the same distance from the mirror. This is a virtual image, as defined earlier.

A flat mirror is placed perpendicular to our field of view. We see the side of the mirror. A bottle is placed to the left of the mirror at distance do to the mirror. Two rays originate from the bottle, strike the mirror, and reflect to an eye that is looking at the mirror. To the right of the mirror, a virtual image of the bottle is included as a backward extrapolation of the path of the rays from the eye to the mirror. The bottle to the right of the mirror is at distance di from the mirror.

Figure 16.5 When two sets of rays from common points on an object are reflected by a flat mirror into the eye of an observer, the reflected rays seem to originate from behind the mirror, which determines the position of the virtual image.

Fun In Physics

Mirror Mazes

Figure 16.6 is a chase scene from an old silent film called The Circus, starring Charlie Chaplin. The chase scene takes place in a mirror maze. You may have seen such a maze at an amusement park or carnival. Finding your way through the maze can be very difficult. Keep in mind that only one image in the picture is real—the others are virtual.

This black-and-white still shot shows Charlie Chaplin and a thief in a maze of mirrors. Images of Chaplin and the thief appear in mirrors, within mirrors, within mirrors.

Figure 16.6 Charlie Chaplin is in a mirror maze. Which image is real?

One of the earliest uses of mirrors for creating the illusion of space is seen in the Palace of Versailles, the former home of French royalty. Construction of the Hall of Mirrors (Figure 16.7) began in 1678. It is still one of the most popular tourist attractions at Versailles.

The Hall of Mirrors at Versailles features an al fresco and gilded domed ceiling; many large, hanging crystal chandeliers; exquisite painted panels on the walls; and mirrors—lots and lots of mirrors—to give an illusion of space. Tourists are pictured roaming the Hall.

Figure 16.7 Tourists love to wander in the Hall of Mirrors at the Palace of Versailles. (credit: Michal Osmenda, Flickr)

Grasp Check

Only one Charlie in Figure 16.8 image is real. The others are all virtual images of him. Can you tell which is real? Hint—His hat is tilted to one side.

Figure 16.8

The virtual images have their hats tilted to the right.
The virtual images have their hats tilted to the left.
The real images have their hats tilted to the right.
The real images have their hats tilted to the left.

Watch Physics

Virtual Image

This video explains the creation of virtual images in a mirror. It shows the location and orientation of the images using ray diagrams, and relates the perception to the human eye.

Play the Virtual Images video.

Compare the distance of an object from a mirror to the apparent distance of its virtual image behind the mirror.

The distances of the image and the object from the mirror are the same.
The distances of the image and the object from the mirror are always different.
The image is formed at infinity if the object is placed near the mirror.
The image is formed near the mirror if the object is placed at infinity.

Some mirrors are curved instead of flat. A mirror that curves inward is called a concave mirror, whereas one that curves outward is called a convex mirror. Pick up a well-polished metal spoon and you can see an example of each type of curvature. The side of the spoon that holds the food is a concave mirror; the back of the spoon is a convex mirror. Observe your image on both sides of the spoon.

Tips For Success

You can remember the difference between concave and convex by thinking, Concave means caved in.

Ray diagrams can be used to find the point where reflected rays converge or appear to converge, or the point from which rays appear to diverge. This is called the focal point, F. The distance from F to the mirror along the central axis (the line perpendicular to the center of the mirror’s surface) is called the focal length, f. Figure 16.9 shows the focal points of concave and convex mirrors.

There are two curved mirrors in this figure. In view (a), the front of a concave mirror is struck by rays of light that originate in front of the mirror. The rays reflect off the concave mirror and converge at varying angles to a point F in front of the mirror. The distance from F to the mirror is f. In view (b), the back of a convex mirror is struck by rays of light that diverge off the back of the mirror at varying angles. If one were to extrapolate the diverging rays backward, to the front of the mirro

Figure 16.9 (a, b) The focal length for the concave mirror in (a), formed by converging rays, is in front of the mirror, and has a positive value. The focal length for the convex mirror in (b), formed by diverging rays, appears to be behind the mirror, and has a negative value.

Images formed by a concave mirror vary, depending on which side of the focal point the object is placed. For any object placed on the far side of the focal point with respect to the mirror, the rays converge in front of the mirror to form a real image, which can be projected onto a surface, such as a screen or sheet of paper However, for an object located inside the focal point with respect to the concave mirror, the image is virtual. For a convex mirror the image is always virtual—that is, it appears to be behind the mirror. The ray diagrams in Figure 16.10 show how to determine the nature of the image formed by concave and convex mirrors.

View (a) shows a concave mirror to the right with a lit candle to the left placed outside the focal point. It is a real image. View (b) shows a concave mirror to the right with a lit candle to the left placed inside the focal point. This image is virtual. View (c) shows a convex mirror to the right with a lit candle to the left. The image is virtual.

Figure 16.10 (a) The image of an object placed outside the focal point of a concave mirror is inverted and real. (b) The image of an object placed inside the focal point of a concave mirror is erect and virtual. (c) The image of an object formed by a convex mirror is erect and virtual.

The information in Figure 16.10 is summarized in Table 16.1.

Type of Mirror	Object to Mirror Distance, d_o	Image Characteristics
Concave	$d_{o} > f$	Real and inverted
Concave	$d_{o} f$	Virtual and erect
Convex	$d_{o} or > f$	Virtual and erect

Table 16.1 Curved Mirror Images This table details the type and orientation of images formed by concave and convex mirrors.

Snap Lab

Concave and Convex Mirrors

Materials

Silver spoon and silver polish, or a new spoon made of any shiny metal

Instructions

Procedure

Choose any small object with a top and a bottom, such as a short nail or tack, or a coin, such as a quarter. Observe the object’s reflection on the back of the spoon.
Observe the reflection of the object on the front (bowl side) of the spoon when held away from the spoon at a distance of several inches.
Observe the image while slowly moving the small object toward the bowl of the spoon. Continue until the object is all the way inside the bowl of the spoon.
You should see one point where the object disappears and then reappears. This is the focal point.

Watch Physics

Parabolic Mirrors and Real Images

This video uses ray diagrams to show the special feature of parabolic mirrors that makes them ideal for either projecting light energy in parallel rays, with the source being at the focal point of the parabola, or for collecting at the focal point light energy from a distant source.

Play the Parabolic Images and Real Images video.

Explain why using a parabolic mirror for a car headlight throws much more light on the highway than a flat mirror.

The rays do not polarize after reflection.
The rays are dispersed after reflection.
The rays are polarized after reflection.
The rays become parallel after reflection.

You should be able to notice everyday applications of curved mirrors. One common example is the use of security mirrors in stores, as shown in Figure 16.11.

The image shows a woman taking a picture in front of a convex mirror.

Figure 16.11 Security mirrors are convex, producing a smaller, upright image. Because the image is smaller, a larger area is imaged compared with what would be observed for a flat mirror; hence, security is improved. (credit: Laura D’Alessandro, Flickr)

Some telescopes also use curved mirrors and no lenses (except in the eyepieces) both to magnify images and to change the path of light. Figure 16.12 shows a Schmidt-Cassegrain telescope. This design uses a spherical primary concave mirror and a convex secondary mirror. The image is projected onto the focal plane by light passing through the perforated primary mirror. The effective focal length of such a telescope is the focal length of the primary mirror multiplied by the magnification of the secondary mirror. The result is a telescope with a focal length much greater than the length of the telescope itself.

There is an eye to the right of the diagram. The eye represents the focal plane. Moving from right to left, in front of the eye, there is a perforated primary mirror and a secondary mirror. The end of the telescope is to the left of the figure. Rays enter the end of the telescoped, hit the primary mirror, bounce backward to the secondary mirror, bounce through the hole (perforation) in the primary mirror, and travel to the eye.

Figure 16.12 This diagram shows the design of a Schmidt–Cassegrain telescope.

A parabolic concave mirror has the very useful property that all light from a distant source, on reflection by the mirror surface, is directed to the focal point. Likewise, a light source placed at the focal point directs all the light it emits in parallel lines away from the mirror. This case is illustrated by the ray diagram in Figure 16.13. The light source in a car headlight, for example, is located at the focal point of a parabolic mirror.

This diagram shows a car headlight, also called a spotlight, which has a light bulb surrounded by a parabolic mirror. The mirror is bowl shaped, with the bulb sitting at the center of recessed side of the bowl. Arrows show that light rays from the bulb extend out in all directions and then bounce off of the mirror so that all of the light rays travel straight out from the headlight

Figure 16.13 The bulb in this ray diagram of a car headlight is located at the focal point of a parabolic mirror.

Parabolic mirrors are also used to collect sunlight and direct it to a focal point, where it is transformed into heat, which in turn can be used to generate electricity. This application is shown in Figure 16.14.

This photo depicts a long length of parabolic trough deflectors angled toward the sun.

Figure 16.14 Parabolic trough collectors are used to generate electricity in southern California. (credit: kjkolb, Wikimedia Commons)

Using a concave mirror, you look at the reflection of a faraway object. The image size changes if you move the object closer to the mirror. Why does the image disappear entirely when the object is at the mirror's focal point?

The height of the image became infinite.
The height of the object became zero.
The intensity of intersecting light rays became zero.
The intensity of intersecting light rays increased.

The Application of the Curved Mirror Equations

Curved mirrors and the images they create involve a fairly small number of variables: the mirror’s radius of curvature, R; the focal length, f; the distances of the object and image from the mirror, d_o and d_i, respectively; and the heights of the object and image, h_o and h_i, respectively. The signs of these values indicate whether the image is inverted, erect (upright), real, or virtual. We now look at the equations that relate these variables and apply them to everyday problems.

Figure 16.15 shows the meanings of most of the variables we will use for calculations involving curved mirrors.

This figure shows an old-fashioned key inside the focal point of a concave mirror. All the variables used for calculations involving curved mirrors depicted.

Figure 16.15 Look for the variables, d_o, d_i, h_o, h_i, and f in this figure.

The basic equation that describes both lenses and mirrors is the lens/mirror equation

\frac{1}{f} = \frac{1}{d_{i}} + \frac{1}{d_{o}} .

This equation can be rearranged several ways. For example, it may be written to solve for focal length.

f = \frac{d_{i} d_{o}}{d_{o} + d_{i}}

Magnification, m, is the ratio of the size of the image, h_i, to the size of the object, h_o. The value of m can be calculated in two ways.

m = \frac{h_{i}}{h_{o}} = \frac{- d_{i}}{d_{o}}

This relationship can be written to solve for any of the variables involved. For example, the height of the image is given by

h_{i} = - h_{o} (\frac{d_{i}}{d_{o}}) .

We saved the simplest equation for last. The radius of curvature of a curved mirror, R, is simply twice the focal length.

R = 2 f

We can learn important information from the algebraic sign of the result of a calculation using the previous equations:

A negative d_i indicates a virtual image; a positive value indicates a real image
A negative h_i indicates an inverted image; a positive value indicates an erect image
For concave mirrors, f is positive; for convex mirrors, f is negative

Now let’s apply these equations to solve some problems.

Worked Example

Calculating Focal Length

A person standing 6.0 m from a convex security mirror forms a virtual image that appears to be 1.0 m behind the mirror. What is the focal length of the mirror?

STRATEGY

The person is the object, so d_o = 6.0 m. We know that, for this situation, d_o is positive. The image is virtual, so the value for the image distance is negative, so d_i = –1.0 m.

Now, use the appropriate version of the lens/mirror equation to solve for focal length by substituting the known values.

Solution

$f = \frac{d_{i} d_{o}}{d_{o} + d_{i}} = \frac{(- 1.0) (6.0)}{6.0 + (- 1.0)} = \frac{- 6.0}{5.0} = - 1.2 m$

Discussion

The negative result is expected for a convex mirror. This indicates the focal point is behind the mirror.

Worked Example

Calculating Object Distance

Electric room heaters use a concave mirror to reflect infrared (IR) radiation from hot coils. Note that IR radiation follows the same law of reflection as visible light. Given that the mirror has a radius of curvature of 50.0 cm and produces an image of the coils 3.00 m in front of the mirror, where are the coils with respect to the mirror?

STRATEGY

We are told that the concave mirror projects a real image of the coils at an image distance d_i = 3.00 m. The coils are the object, and we are asked to find their location—that is, to find the object distance d_o. We are also given the radius of curvature of the mirror, so that its focal length is f = R/2 = 25.0 cm (a positive value, because the mirror is concave, or converging). We can use the lens/mirror equation to solve this problem.

Solution

Because d_i and f are known, the lens/mirror equation can be used to find d_o.

16.1

\frac{1}{f} = \frac{1}{d_{i}} + \frac{1}{d_{o}}

Rearranging to solve for d_o, we have

16.2

d_{o} = \frac{d_{i} f}{d_{i} - f} .

Entering the known quantities gives us

16.3

d_{o} = \frac{(3.00 \times 10^{2}) (25.0)}{(3.00 \times 10^{2}) - 25.0} = 27.3 cm .

Discussion

Note that the object (the coil filament) is farther from the mirror than the mirror’s focal length. This is a case 1 image (d_o > f and f positive), consistent with the fact that a real image is formed. You get the most concentrated thermal energy directly in front of the mirror and 3.00 m away from it. In general, this is not desirable because it could cause burns. Usually, you want the rays to emerge parallel, and this is accomplished by having the filament at the focal point of the mirror.

Note that the filament here is not much farther from the mirror than the focal length, and that the image produced is considerably farther away.

Practice Problems

A concave mirror has a radius of curvature of

0.8 m

. What is the focal length of the mirror?

$- 0.8 m$
$- 0.4 m$
$0.4 m$
$0.8 m$

What is the focal length of a makeup mirror that produces a magnification of 1.50 when a person’s face is 12.0 cm away? Construct a ray diagram using paper, a pencil and a ruler to confirm your calculation.

–36.0 cm
–7.20 cm
7.20 cm
36.0 cm

Check Your Understanding

Exercise 1

How does the object distance, d_o, compare with the focal length, f, for a concave mirror that produces an image that is real and inverted?

d_o > f, where d_o and f are object distance and focal length, respectively.
d_o < f, where d_o and f are object distance and focal length, respectively.
d_o = f, where d_o and f are object distance and focal length, respectively.
d_o = 0, where d_o is the object distance.

Exercise 2

Use the law of reflection to explain why it is not a good idea to polish a mirror with sandpaper.

The surface becomes smooth, and a smooth surface produces a sharp image.
The surface becomes irregular, and an irregular surface produces a sharp image.
The surface becomes smooth, and a smooth surface transmits light, but does not reflect it.
The surface becomes irregular, and an irregular surface produces a blurred image.

Exercise 3

An object is placed in front of a concave mirror at a distance that is greater than the focal length of the mirror. Will the image produced by the mirror be real or virtual? Will it be erect or inverted?

It is real and erect.
It is real and inverted.
It is virtual and inverted.
It is virtual and erect.

16.1 Reflection

16.1 Reflection

Learning Objectives

Learning Objectives

Characteristics of Mirrors